Return to Problems

Before answering questions #12 through 14, we need to enter the data into our calculator.

Press the [ON] button.

Press [MODE] once and you should see:

You calculator is always in one of these modes (COMP, SD, or REG).

• "REG" is the regression mode and is used for two-variable statistics (called "bivariate statistics"). To select "REG" from the first menu, you press 3.
  Press [3]
  Then you are faced with a secondary menu:

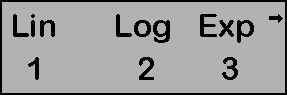
Press [1] (we will only do linear regressions).

You should see a very small REG near the middle bottom of the screen - just above the [REPLAY] buttons.

Before we begin, I want you to locate the data entry key [M+] on your calculator. It is the button just above the red [AC] button. I also want you to note where the [,] button is. It is directly above the red [DEL] button. It is also important that you do not confuse the negative button [(-)] on the left side of your calculator with the subtraction button which is on the lower right side of your calculator. The negative button [(-)], just above the [STO] button, is used to enter negative numbers. The subtraction button [–], just above the [=] button, is not used for data entry.

The basic unit in bivariate algebra, bivariate geometry, and bivariate statistics is a "point." A point is specified by two variables, an x-value and a y-value. Points are written in the form of (x, y). For example, (79, 15) is a point with an x-value of 79 and a y-value of 15.

This section assumes your calculator is in Regression mode.
[SHIFT] [AC] [=] to clear your statistical memory.
Your screen will show SCL for "Statistical Clear." Don't worry, this will disappear when we start entering data. If your calculator is does not show SCL, repeat this process until it does. Let's enter that data now:

[7] [9] [,] [1] [5] [M+] entered the first point.
[9] [6] [,] [2] [5] [M+] entered the second point.
[7] [6] [,] [1] [1] [M+]
[6] [6] [,] [2] [3] [M+]
[8] [5] [,] [2] [4] [M+]
[7] [2] [,] [1] [7] [M+]
[9] [0] [,] [1] [8] [M+]
[7] [3] [,] [1] [5] [M+]
[6] [8] [,] [1] [9] [M+]
[8] [0] [,] [1] [9] [M+] entered the last point.

That's it!! Wasn't that easy? We only need to enter the data once, and as long as we do not clear our calculator, we can ask our calculator to compute a number of different statistics (or parameters) based on that data. Do not clear your calculator until after you have finished problem number 14.

12. What is the correlation coefficient (r) for the data in Data Set B? Does this differ significantly from rho (ρ) = 0 at alpha (α) = .05 (two-tailed)? Do a complete hypothesis test.

Step 1:

H₀: rho (ρ) = 0; that is, our sample came from a population in which there is no linear relationship between the x variable and the y variable.

Step 2:

H₁: rho (ρ) 0; that is, our sample came from a population in which there is a linear relationship between the x variable and the y variable.

Step 3:

alpha level: alpha (α) = .05

Step 4:

Rejection Rule: Reject H0 if |r_comp| > .632
To obtain the r_crit, we must determine our degrees of freedom. Df equals the number of data points (pairs of coordinates) minus two. We have 10 data points giving us 8 df. To find our r_crit, we look in Table E on page 408 of our text. We go to the intersection of 8 df and 5% to obtain our critical value of .632.

Step 5:

Computation: Because we have already entered the data into our calculator, this will be easy. The symbol for the Pearson Product Moment Correlation Coefficient is r. To find the r for our data, press:
[SHIFT] [(] [=] your display should read 0.337438136. Therefore, r = .337438136.

Step 6:

Decision: Because |0.3374| is not greater than .632, we fail to reject H₀.

Step 7:

Conclusion: There is not enough evidence to conclude that there is a relationship between x and y. There is not a significant relationship between our x values and y values.

Return to Problems